Hint for Section 10.2 Question 1
1. Recall that if a relation R on a set A is
specified as a list of ordered pairs then:
R is reflexive if, and only if,
the ordered pair (x, x) is an element of R, for all x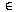
A.
R is symmetric if, and only if,
whenever (x, y)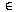R then the ordered
pair (y, x) also belongs to R.
R is transitive if, and only if,
whenever (x, y)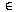R and (y, z)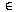R then the ordered pair (x, z) also
belongs to R.
If you find it difficult to keep track of the
ordered pairs, you might like to draw the directed graphs for each relation and then
determine reflixivity, symmetry and transitivity from the graph.
Back to Section 10.2
Full solution